おわんとおはしの問題 - 科学のおもちゃ箱@Hatena

OKWave>http://okwave.jp/qa/q5962844.html より。つりあいの問題としては見かけるが，その微小振動までを考察する。
【問題】
長さ $3a$ の棒(質量 $m$ )が半球面(半径 $a$ )の中にある。棒の一端が球の中にあり、半球の縁に支えられている。重力加速度の大きさを $g$ とし，摩擦は無視できるものとする。
f:id:yokkun831:20190322090850j:plain

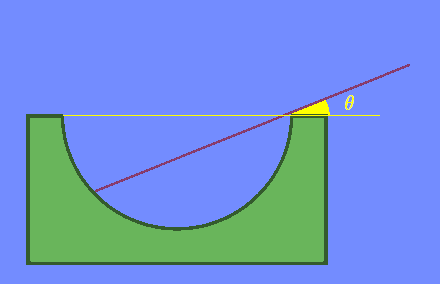
(1) つりあいの状態における，棒の水平方向からの角度 $\theta_0$ を求めよ。

(2) つりあいの状態からずれた場合に生じる，鉛直面内の微小振動の周期を求めよ。

※Algodooの設定は， $a=48$ [m]という巨大なものである。

【解答】
(1)
f:id:yokkun831:20190322091338j:plain
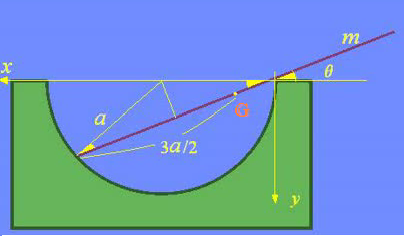
(2)への発展のために，力のつりあいからでなく，位置エネルギーを最小とする位置を求める。

棒と半球の接点を原点に，水平方向に $x$ 軸，鉛直下方に $y$ 軸をとる。棒の重心の座標・速度成分は，水平方向からの角度 $\theta$ として

$x = \left(2a \cos\theta - \displaystyle\frac{3}{2}a\right)\cos\theta$

$y = \left(2a \cos\theta - \displaystyle\frac{3}{2}a\right)\sin\theta$

$\dot{x} = a\dot{\theta}\sin\theta\left(\displaystyle\frac{3}{2} - 4\cos\theta\right)$

$\dot{y} = a\dot{\theta}\left(-2\sin^2\theta+2\cos^2\theta-\displaystyle\frac{3}{2}\cos\theta\right)$

速さを $v$ として，

$v^2 = \dot{x}^2 + \dot{y}^2 = a^2\dot{\theta}^2\left(\displaystyle\frac{25}{4} - 6\cos\theta\right)$

運動エネルギー，位置エネルギーは，

$K = \displaystyle\frac{1}{2}mv^2 + \frac{1}{2}I\dot{\theta}^2 = ma^2\dot{\theta}^2\left(\frac{7}{2} - 3\cos\theta\right)\quad , \quad I = \frac{1}{12}m(3a)^2$

$U = -mgy = -mga\left(2\cos\theta - \displaystyle\frac{3}{2}\right)\sin\theta$

となる。

$\displaystyle\frac{dU}{d\theta} = mga\left\{2\sin^2\theta - \left(2\cos\theta - \frac{3}{2}\right)\cos\theta\right\} = -\frac{1}{2}mga(8\cos^2\theta - 3\cos\theta - 4)$

$\theta=\theta_0$ において，これがゼロになるから，

$\cos\theta_0 = \displaystyle\frac{3+\sqrt{137}}{16}$

を得る。

(2)

ラグランジアンから運動方程式を導出して近似してもよいが，より簡明と思われるエネルギー保存を用いる。

エネルギーは，

$E = ma^2\theta^2\left(\displaystyle\frac{7}{2} - 3\cos\theta\right) - mga \sin\theta\left(2\cos\theta-\displaystyle\frac{3}{2}\right)$

つりあい位置　 $\theta_0$ からの微小角変位 $\phi$ とすると $\theta=\theta_0+\phi$ 。

最大運動エネルギー＝初期位置とつりあい位置の位置エネルギー差

であるから，

$ma^2{\dot{\phi}_{\rm max}}^2\left(\displaystyle\frac{7}{2} - 3\cos\theta_0\right)$

$= -mga\Big[\sin(\theta_0+\phi_{\rm max})\left\{2\cos(\theta_0+\phi_{\rm max})-\displaystyle\frac{3}{2}\right\} - \sin\theta_0\left(2\cos\theta_0-\displaystyle\frac{3}{2}\right)\Big]$

右辺を $\phi_{\rm max}$ について展開すると，つりあい条件から $\phi_{\rm max}$ の１次項は消えるので，２次項まで残して近似する。

$\displaystyle\frac{1}{2}\cdot{\dot{\phi}_{\rm max}}^2 = \frac{1}{2}\cdot\frac{g}{a}\cdot\frac{\displaystyle 4\sin 2\theta_0 - \frac{3}{2}\sin\theta_0}{7 - 6\cos\theta_0}\cdot\phi_{\rm max}^2$